To do this, you can determine how much each angle measures using the cosine law, which is a formula that relates the angles to the sides of any triangle.
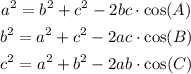
For example, to first find the measure of angle A, you have

Similarly, you can find the angle B
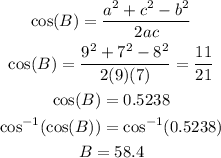
To find the angle C you can use the following expression that indicates that the sum of the internal angles of a triangle is 180
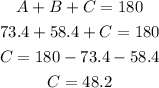
Therefore, the order of the angles from largest to smallest is