Step-by-step explanation:
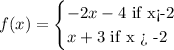
The line of the first part of the function, without the restriction of x <=-2:
The line of the second part of the function, without the restriction is:
To graph the full function, we have to draw a line over the green dashed line until x = -2 and then continue drawing but now over the orange dashed line
Answer:
The graph of the function is:
• Domain:
![\begin{gathered} (-\infty,\infty) \\ \text{ or also} \\ -\infty• Range[tex]\begin{gathered} \lbrack0,\infty) \\ \text{ or also} \\ 0\le y<\infty \end{gathered}]()