Solution
- The equation given is a quadratic equation and the graph of a quadratic equation has either a minimum or a maximum never both.
- The equation given is
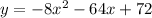
- This equation has a maximum value because:
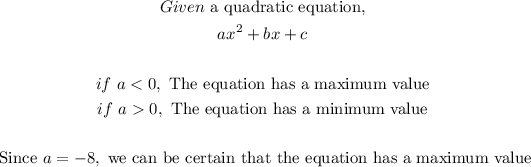
- The maximum value happens to coincide with the vertex of the equation. The x-value for this vertex is gotten using the formula below:
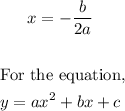
- Thus, we can find the x-value of the vertex using the above formula. Once we have the x-value of the vertex, we can simply substitute the x-value into the equation to get the corresponding y-value which would represent the highest possible y-value.
- Thus, we have:

Final Answer
The highest possible y-value is y = 200