Given
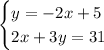
To solve the equation system you can use the substitution method. The first equation is expressed for y, you can replace it with the second equation, this way you will be able to determine an equation with one unknown "x"
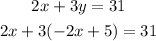
To solve it for x, first, you have to distribute the multiplication on the parentheses term:
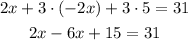
Second, simplify the like terms, and pass the constants to the right side of the equation by applying the opposite operation to both sides of it:
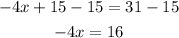
Third, divide both sides of the equation by -4 to determine the value of x
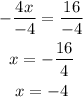
Once you have calculated the value of x, replace it into the first equation to determine the corresponding value of y:
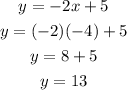
The solution for this equation system is (-4,13)