Question

A triangular prism has a base of 6cm, 8cm, and 10cm long. Its lateral area is 744cm?.What is the height of the solid?

Answer 1

We have the following equation for the lateral surface area of a triangular prism:

`A=h(a+b+c)`

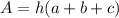
where a,b and c are the measures of the sides of the triangular base.

In this case, we have the following:

`\begin{gathered} A=744 \\ a=6 \\ b=8 \\ c=10 \end{gathered}`

using the formula, we get:

`744=h(6+8+10)`

now, we can solve for h to find the height:

`\begin{gathered} h(6+8+10)=744 \\ \Rightarrow24h=744 \\ \Rightarrow h=(744)/(24)=31 \\ h=31 \end{gathered}`

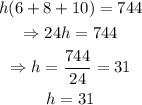
therefore, the height of the solid is 31 cm