Question

find the equation of the line perpendicular to y=1/2x-5 that passes through the point (2,7) write this in slope intercept form.

Answer 1

The slope-intercept form of a line is:

`y=mx+b`

Where m is the slope of the line and b is the y-intercept.

If two lines are perpendicular, their slopes are the inverse multiplied by (-1)

If a line has a slope of m, then the slope of a line perpendicular to it is:

`Slope\text{ }perpendicular=-(1)/(m)`

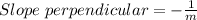
We know that the slope of the perpendicular line is 1/2, then the slope of the line we are calculating is:

`m=-(1)/((1)/(2))=-2`

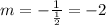
Now, we can use the point-slope form of a line. Given a point P and a slope m, the equation of the line with slope m that passes through the point P is:

`\begin{gathered} P=(x_P,y_P) \\ . \\ y=m(x-x_P)+y_P \end{gathered}`

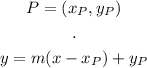
In this case, the slope is m = -2 and passes through the point P = (2, 7)

We write:

`y=-2(x-2)+7`

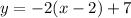
If we simplify this expression we get the equation of the line in slope-intercept form:

`y=-2x-2(-2)+7=-2x+4+7=-2x+11`

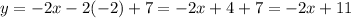
Thus, the answer is:

`y=-2x+11`