We have to perform an hypothesis test for the mean.
The claim is that the patient's mean HC is greater than the population average.
Then, the null and alternative hypothesis are:
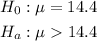
The significance level is 0.05.
The sample has a size n = 10.
The sample mean is M = 15.6.
The standard deviation of the population is known and has a value of σ = 2.5.
We can calculate the standard error as:
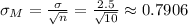
Then, we can calculate the z-statistic as:
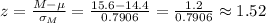
This test is a right-tailed test, so the P-value for this test is calculated as:
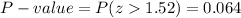
As the P-value (0.06) is bigger than the significance level (0.05), the effect is not significant.
The null hypothesis failed to be rejected.
At a significance level of 0.05, there is not enough evidence to support the claim that the patient's mean HC is greater than the population average.
Answer:
z = 1.52
P-value = 0.064
Conclusion: fail to reject the null hypothesis.