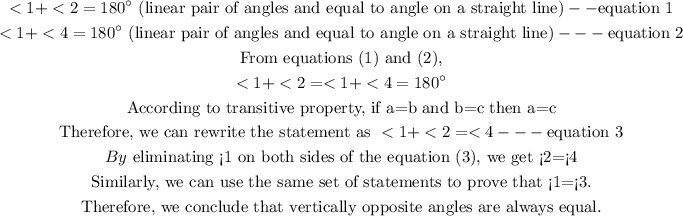
SOLUTION
Given the question in the question tab, the following are the solution steps to prove the theorem
STEP 1: Write the theorem
Theorem: Vertical Angles are congruent
Step 2: Define Vertical Angles
When two lines intersect, four angles are formed. There are two pairs of nonadjacent angles. These pairs are called vertical angles. In the image given below, (∠1, ∠3) and (∠2, ∠4) are two vertical angle pairs.
STEP 3: State the Vertical angles theorem
Vertical angles theorem or vertically opposite angles theorem states that two opposite vertical angles formed when two lines intersect each other are always equal (congruent) to each other.
STEP 4: Prove the theorem
The proof is based on straight angles. We already know that angles on a straight line add up to 180°.