Question

Jan Reus signed a promissory note for $15,000 at 7.5% interest that resulted in interest charges of $550. Find the term of the note to the nearest day

Answer 1

For us to be able to determine the term of the note, we will be using the Simple Interest Formula:

`\text{ Simple Interest = }\frac{\text{PRT}}{100}`

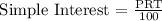
Where,

P = Principal Amount

R = Interest Rate

T = Time (Terms in years)

We get,

P = $15,000

R = 7.5%

Simple Interest = $550

Let's find T in days,

`\text{ Simple Interest = }\frac{\text{PRT}}{100}`
`\text{ 550 = }\frac{15,000\text{ x 7.5 x T}}{100}`
`\text{ 550 = 1125T}`
`\text{ 1125T = 550}`
`\text{ }\frac{\text{1125T}}{\text{ 1125}}\text{ = }\frac{\text{550}}{\text{ 1125}}`
`\text{ T = }\frac{\text{ 22}}{\text{ 45}}\text{ Years = }\frac{\text{ 22}}{\text{ 45}}\text{ (years) x }\frac{\text{ 365 days}}{\text{ 1 (year)}}`
`\text{ T = }\frac{\text{ 22 x 365}}{\text{ 45}}\text{ days = }\frac{\text{ 8,030}}{\text{ 45}}\text{ days}`
`\text{ T = 178.4}4\text{ }\approx\text{ 178 days}`

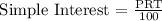
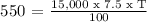
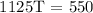
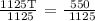
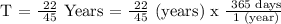
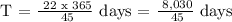
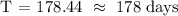
Therefore, the term of the note is approximately 178 days.