Question

State how you know they are similar, then find the scale factor from the small to large triangle, then find x.

Answer 1

The two triangles are similar because all the corresponding angles of both triangles are congruent.

To get the scale factor from small to large triangle, the formula is:

`SF=\frac{size\text{ of the large triangle}}{\text{size of the small triangle}}`

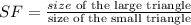
In the small triangle, we have a side that is 5 units long. In the large triangle, the corresponding side is 7 units long. Let's plug these in to the formula above.

`SF=(7)/(5)=1.4`

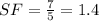
The scale factor is 7/5 or 1.4.

To determine the value of x, we can still use the formula above.

In the small triangle, we have a side that is 10 units long. In the large triangle, the corresponding side is 10 + x units long. Let's plug these into the scale factor formula.

`\begin{gathered} 1.4=(10+x)/(10) \\ 14=10+x \\ x=14-10 \\ x=4 \end{gathered}`

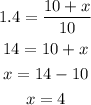
Therefore, the value of x is 4.