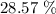Answer:
The increased price must be decreased by 28.57%
Step-by-step explanation:
Given that the price of pulses has increased by 40%.
Let x reprsent the initial price.
and f the increased price;

To bring the price back to x we want to calculate the percentage decrease;
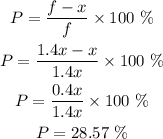
Therefore, the increased price must be decreased by 28.57%