Question

Hi, can you help me to solve thisexercise, please!!For cach polynomial, LIST all POSSIBLE RATIONAL. ROOTS•Find all factors of the leading coefficient and constant value of polynonnal.•ANY RATIONAL ROOTS =‡ (Constant Factor over Leading Coefficient Factor)4x^2-9

Answer 1

Rational roots: -3/2 and 3/2

Step-by-step explanation

The leading coefficient is the value that multiplies the variable with the greatest exponent. In this case, the leading coefficient is 4.

To find the roots, we have to solve,

`4x^2-9=0`

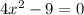
For that, we add the constant factor 9 to both sides,

`\begin{gathered} 4x^2-9+9=0+9 \\ \\ 4x^2=9 \end{gathered}`

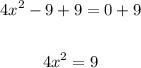
Divide both sides by the leading coefficient,

`\begin{gathered} (4x^2)/(4)=(9)/(4) \\ \\ x^2=(9)/(4) \end{gathered}`

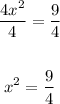
And take the square root of both sides.

`\begin{gathered} \sqrt[]{x^2}=\pm\sqrt[]{(9)/(4)} \\ \\ x=\pm(3)/(2) \end{gathered}`

Hence, all the rational roots of this polynomial are -3/2 and 3/2.