Answer:
The population function is given below as

Step 1:
To figure out the initial price of the item, we will substitute the value of t=0
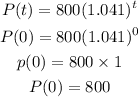
Hence,
The initial pice of the item is = $800
Step 2:
To determine if the function represents a growth or decay, we will use the relation below
The function for exponential growth is given below as
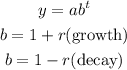
By comparing coefficients, we can see that
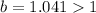
Hence,
The function represents GROWTH
STEP 3:
To figure out the percentage of price change each year, we will use the formula below
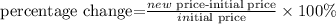
To figure out the thenew price, we will substitute the value of t=1
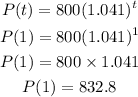
By substituting the values, we will have the percentage to be
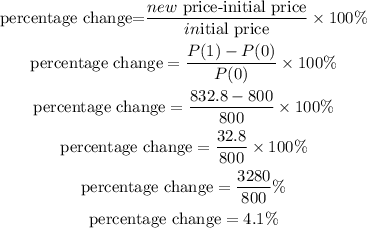
Hence,
The percentage = 4.1%