Question

6) Fadel owns a small business that manufactures and sells clocks. The clocks cost $12.50 each to manufacture with a monthly overhead of $4500. Fadel sells the clocks for $37.50 each (5pts each) a) Analyze and set-up a cost equation and a revenue equation for these clocks. b) Find the cost and how many clocks Fadel needs to make and sell to break-even. 7) Sia owns a candy store, and she wants to mix gummy worms worth $3 per pound with gummy bears worth $1.50 per pound to make 30 pounds of a mixture worth $63.00. (Spts each) a) Analyze and set-up a system of equations for this mixture (quantity-value system). b) Solve this system of equations to determine how many pounds of each of candy Sia should use for this mixture.

Answer 1

`\begin{cases}w+b=30 \\ 3w+1.5b=63\end{cases}`

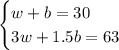
Solution: w = 12 , b = 18

Step-by-step explanation:

Part A;

Let us call

w = # of lb of gummy worms

b = # of lb of gummy bears

We are told that one point of gummy worms costs $3, meaning w pounds cost 3 *w. Moreover, one pound of gummy worms costs $1.5, meaning b pounds ost 1.5 * b.

The total worth of the gummies is $63; therefore,

`3w+1.5b=63`

Also, we know that the total number of pounds of gummies is 30 pounds; therefore,

`w+b=30`

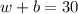
Hence, we have the system

`\begin{cases}w+b=30 \\ 3w+1.5b=63\end{cases}`

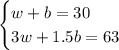
Part B:

To solve the system given above, we first solve for w in the first equation.

`w+b=30`
`\rightarrow w=30-b`

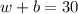
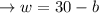
We put the value of the w given above into the second equation to get

`\begin{gathered} 3w+1.5b=63 \\ \rightarrow3(30-b)+1.5b=63 \end{gathered}`
`=90-3b+1.5b=63`
`90-1.5b=63`

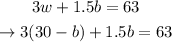
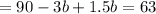
subtracting 90 from both sides gives

`-1.5b=-27`

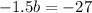
Finally, dividing both sides by -1.5 gives

`\boxed{b=18}`

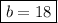
With the value of b in hand, we now put it into w + b = 30 and solve for w to get

`w+18=30`
`w=30-18`
`\boxed{w=12}`

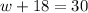
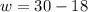
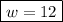
Hence, the solution to the system is w = 12, b = 18.