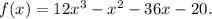Step-by-step explanation
We must find the equation of a polynomial with the following zeros: -5/4, -2/3 and 2.
(1) The general equation for this polynomial is:

Where x₁, x₂ and x₃ are the zeros.
(2) Replacing the zeros from above, we have:
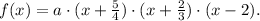
(3) Expanding the last expression, we have:
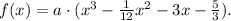
(4) Comparing the last expression with the possibilities that we have as answers, we see that a = 12. Replacing this number in the expression above, we get:
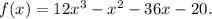
Answer
D.