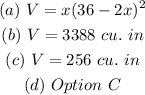Question

Please help me solve this fast and don’t forget the last one

Answer 1

Explanation

We are given the following information:

A square-piece of cardboard is used to form a box as shown in the image below:

Therefore, we have:

- For Part A:

`\begin{gathered} \text{ The box is formed when x-inches is cut-off, so} \\ Side\text{ }of\text{ }the\text{ }box=(36-x-x)=(36-2x) \\ Depth\text{ }of\text{ }the\text{ }box=x \\ \\ \therefore Volume=x(36-2x)^2 \end{gathered}`

- For Part B:

`\begin{gathered} V=x(36-2x)^2 \\ where \\ x=7 \\ \\ \therefore V=7(36-2\cdot7)^2 \\ V=7(36-14)^2 \\ V=7(22)^2 \\ V=3388\text{ }cu.\text{ }in \end{gathered}`

- For Part C:

`\begin{gathered} V=x(36-2x)^2 \\ where \\ x=16 \\ V=16(36-2\cdot16)^2 \\ V=16(36-32)^2 \\ V=16(4)^2 \\ V=256\text{ }cu.\text{ }in \end{gathered}`

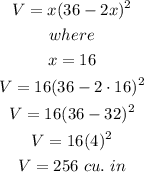
- For Part D:

Using a graphing calculator, we have the graph to be:

Option C is correct.

Hence, the answers are:

`\begin{gathered} (a)\text{ }V=x(36-2x)^2 \\ (b)\text{ }V=3388\text{ }cu.\text{ }in \\ (c)\text{ }V=256\text{ }cu.\text{ }in \\ (d)\text{ }Option\text{ }C \end{gathered}`