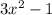Given the functions below
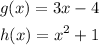
To find the composite function g(h(x)), we will substitute the whole of h(x) as x in the function g(x). This process can be done below
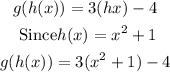
Simplify the equation above using distributive property of algebra

Hence, the composite function g(h(x)) is