Question

What is the value of tan0 in the unit circle below? (see the attached picture)

Answer 1

Step-by-step explanation: To solve this question first we need to know that the terminal point pair (little red point in the image) represented by the coordinates represent the (cosine, sine) as shown bellow

We also need to understand that the "tan" formula can be expressed as follows

`tan\theta=(sin\theta)/(cos\theta)`

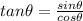
So all we need to do is to substitute and calculate.

Step 1: Let's substitute the values and calculate as follows

`tan\theta=(sin\theta)/(cos\theta)=((1)/(2))/((√(3))/(2))=(1)/(2)*(2)/(√(3))=(2)/(2*√(3))=(1)/(√(3))`

Step 2: Now let's rationalize the result as follows

`tan\theta=(1)/(√(3))=(1)/(√(3))*(√(3))/(√(3))=(√(3))/(3)`

Final answer: So the final answer is

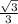
`(√(3))/(3)`

.