Note that :
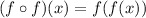
The composition of f of f of x is the same as shown above.
From the given problem, we have :
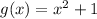
g(g(x)) will be the function which g(x) substitutes the value of x in g(x), this will be :
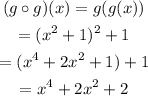
The answer for (g o g)(x) = x^4 + 2x^2 + 2
The next function is :
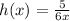
Same as the method we used above.
(h o h)(x) is the same as h(h(x)), and this will be :
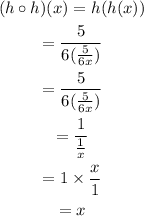
Therefore, the answer for (h o h)(x) = x