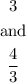Question

The sum of a number and 4 times it’s reciprocal is 13/3. Find the number(s).

Answer 1

Let the unknown number be "x"

We will write an algebraic equation from the word problem given. Then we will solve for "x".

Given,

Sum of number (x) and 4 times the reciprocal is 13/3

We can convert it into an algebraic equation:

`x+(4*(1)/(x))=(13)/(3)`

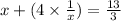
Now, let's solve for the unknow, x,

`\begin{gathered} x+(4*(1)/(x))=(13)/(3) \\ x+(4)/(x)=(13)/(3) \\ (x^2+4)/(x)=(13)/(3) \\ 3(x^2+4)=13* x \\ 3x^2+12=13x \\ 3x^2-13x+12=0 \\ (x-3)(x-(4)/(3))=0 \\ x=3 \\ x=(4)/(3) \end{gathered}`

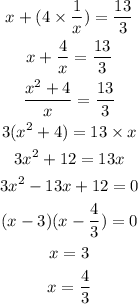
The numbers are

`\begin{gathered} 3 \\ \text{and} \\ (4)/(3) \end{gathered}`