The Solution:
It is given that
For Bank A:
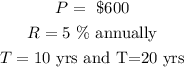
For Bank B:
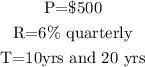
By the formula for the compound interest, we have
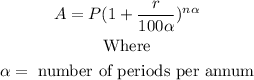
For Bank A:

Substituting the corresponding values, we have
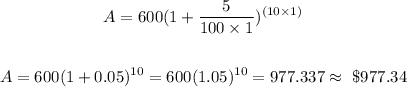
For Bank A for after 20 years:
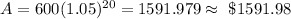
Similarly, for Bank B:
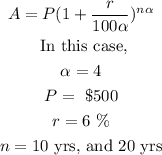
Substituting these values in the formula, we get
![undefined]()