In order to simplify this expression, first let's put every quadratic polynomial in the factored form:

So, switching the division into a multiplication and inverting the second fraction, we have:
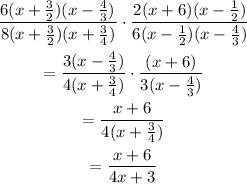
Therefore the numerator is x + 6 and the denominator is 4x + 3.