Question

The answer simplifying this problem please. With positive exponents only.

Answer 1

1/(xy²)

Step-by-step explanation:

The given expression is

`(x^(-4)y^(-2)z^7)/(x^(-3)z^7)`

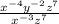
To simplify the expression, we will use the following properties

`\begin{gathered} (x^a)/(x^b)=x^(a-b) \\ x^(-a)=(1)/(x^a) \end{gathered}`

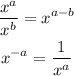
So, we need to apply the first property for x and z to get:

`\begin{gathered} (x^(-4)y^(-2)z^7)/(x^(-3)z^7)=x^(-4-(-3))y^(-2)z^(7-7)^{} \\ ^{}=x^(-4+3)y^(-2)z^0 \\ ^{}=x^(-1)y^(-2)\cdot1 \\ =x^(-1)y^(-2) \end{gathered}`

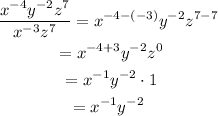
Because any number to the power of 0 is equal to 1. Then, apply the second property to get

`(1)/(x^1y^2)=(1)/(xy^2)`

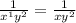
Therefore, the answer is 1/(xy²)