For the information given in the statement and in the graph you have
*Point T has coordinates:

*Point R has coordinates:
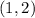
*Point S has coordinates:

Now to find the slopes of the segments you can use the slope formula, that is
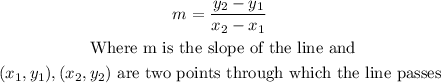
So, the slope of TR is
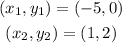
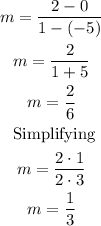
Now, the slope of RS is

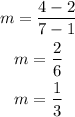
Now, the slope of TS is
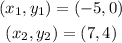

For point 7, you know that a single line passes through two points, and since the segments, TR, RS, and TS have the same slope, that is, 1/3 then the SLOPE of the line is 1/3.
For point 8, you know that the constant rate of change with respect to the variable x of a linear function is the slope of its graph. Therefore, the CONSTANT rate of change is 1/3.