2)
x=-1
y=-1
Step-by-step explanation
when you have a system of 2 equations and 2 variables ( x and y ), to solve the systme graph each line and the point where the lines intersect each other is the solution
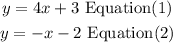
Step 1
graph equation 1 (green)
find two points
a) when x= 0
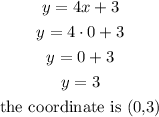
b) when x= 2
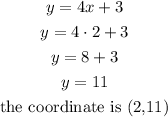
now, draw a line that passes thougth the points (0,3) and (2,11)
Step 2
now, do the same for equation (2)(blue)
when x=0

when x=2
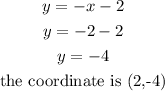
now, draw a line that passes thougth the points (0,-2) and (2,-4).(blue line)
the answer is the point where the lines intersect each other so, the answer is
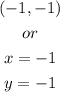
I hope this helps you