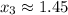Given the equation:
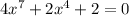
You need to remember that Newton's method to approximate a root of the equation provides this formula:
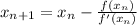
In this case:

Then, you need to derivate it, in order to find:

Use these Derivative Rules:
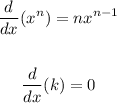
Where "k" is a constant.
You get:
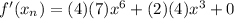
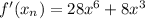
• Knowing that:
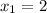
You can set up that:
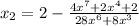
Substitute the given value of "x" and evaluate, in order to find the second approximation:
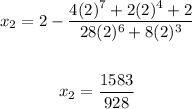
• Now you can set up that:
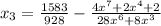
Then, substituting the corresponding x-value and evaluating, you get:
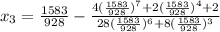
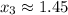
Hence, the answers are:
• Second approximation:
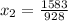
• Third approximation: