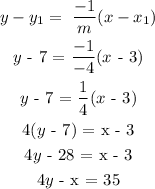Answer:
4y - x = 35
Explanations:
The given points are A(3, 7) and B(2, 11)
The general equation of a line with the points A(x₁ , y₁) and B(x₂ , y₂) is :
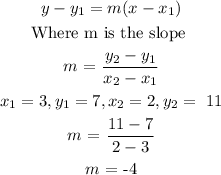
Putting these values into the general line equation:
For the line parallel to the lines formed by the points slope = -1 / m
Slope = -1 / 4