Question

graphically determine the slopes of the line segments that create `ACD. Write your final slopes in simplest form.

Answer 1

`\begin{gathered} \text{Slope}_(AD)=(9)/(11) \\ \text{Slope}_(CD)=(1)/(3) \\ \text{Slope}_(AC)=(7)/(2) \end{gathered}`

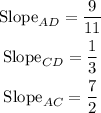
Step-by-step explanation

The slope of a line is a measure of its steepness,it is change in y over change in x

`\text{slope}=\frac{change\text{ in y}}{\text{change in x}}`

Step 1

AD

so, check in the graph for the change in y and the change in x ( count the units)

as we can see:

change in y(green)= 9

change in x(red)=11

therefore

`\begin{gathered} \text{Slope}_(AD)=\frac{change\text{ in y }}{\text{change in x}} \\ \text{Slope}_(AD)=(9)/(11) \end{gathered}`

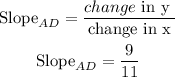
Step 2

now, do the same for segment CD

`\begin{gathered} \text{Slope}_(CD)=(5)/(15)=(1)/(3) \\ \text{Slope}_(CD)=(1)/(3) \end{gathered}`

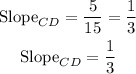
Step 3

finally, segment AC

`\begin{gathered} \text{Slope}_(AC)=\frac{change\text{ in y(purple) }}{\text{change in }x(\text{yeloow)}} \\ \text{Slope}_(AC)=(14)/(4)=(7)/(2) \\ \text{Slope}_(AC)=(7)/(2) \end{gathered}`

I hope this helps you