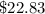Answer:
The monthly cost for 85 minutes of call is;
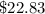
Step-by-step explanation:
Given that the monthly cost (in dollars) of a long-distance phone plan is a linear function of the total calling time (in minutes).
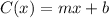
The monthly cost for 43 minutes of calls is $18.21 ;
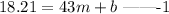
the monthly cost for 102 minutes is $24.70;
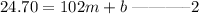
to get m and b, subtract equation 1 from 2;
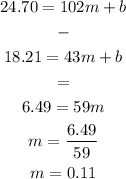
to get b, substitute the value of m into equation1;
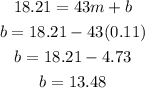
So, the linear function that can represent the monthly cost of phone plan is;
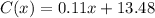
For the monthly cost for 85 minutes of call, we have;
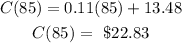
Therefore, the monthly cost for 85 minutes of call is;