Given data
*The speed of the cyclist is u = 7 m/s
*The given distance is s = 11 m
*The given time is T = 0.400 s
(a)
The formula for the acceleration of the cyclist to just avoid the collision is given by the equation of motion as
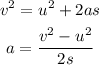
*Here v = 0 m/s is the final speed of the cyclist
Substitute the known values in the above expression as
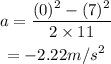
Hence, the acceleration of the cyclist to avoid the collision is a = -2.22 m/s^2
(b)
The formula for the time taken to cross the distance of 11 m is given by the equation of motion
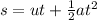
Substitute the known values in the above expression as
![undefined]()