Question

Can I get a tutor to help me with this ?

Answer 1

Okay, here we have this:

Considering the provided infotmation, we are goning to calculate the requested time, so we obtain the following:

So we will set the function equal to zero and solve for t, we have:

`\begin{gathered} ((1)/(2))32t^2+16t=480 \\ ((1)/(2))32t^2+16t-480=0 \\ 16t^2+16t-480=0 \\ t_(1,2)=(-16\pm√(16^2-4(16)(-480)))/(2(16)) \\ t_(1,2)=(-16\pm√(16^2+30720))/(32) \\ t_(1,2)=(-16\pm√(256+30720))/(32) \\ t_(1,2)=(-16\pm176)/(32) \\ t_1=(-16+176)/(32),t_2=(-16-176)/(32) \\ t_1=(160)/(32),t_2=(-192)/(32) \\ t_1=5,t_2=-6 \end{gathered}`

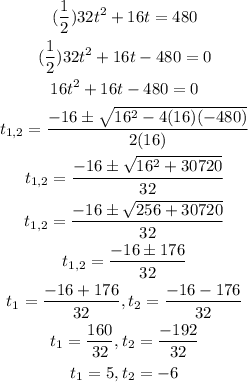
Finally we obtain that The ball will take 5 seconds to hit the ground.