ANSWER
![480,000\operatorname{mm}^3]()
Step-by-step explanation
We want to find the volume of 20 L-shaped erasers.
First, we have to find the volume of one eraser. To do this, we have to partition the eraser into two rectangular prisms:
To find the volume of the prism, apply the formula:
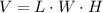
where L = length; W = width; H = height
Therefore, for A, we have:
![\begin{gathered} V_A=60\cdot20\cdot10 \\ V_A=12,000\operatorname{mm}^3 \end{gathered}]()
And for B, we have:
![\begin{gathered} V_B=60\cdot20\cdot10 \\ V_B=12,000\operatorname{mm}^3 \end{gathered}]()
The volume of the shape is the sum of the volumes of the partitions. Hence, the volume of the shape is:
![\begin{gathered} V=V_A+V_B_{} \\ V=12,000+12,000 \\ V=24,000\operatorname{mm}^3 \end{gathered}]()
Hence, a pack of 20 will have a volume of:
![\begin{gathered} V=20\cdot24,000 \\ V=480,000\operatorname{mm}^3 \end{gathered}]()
That is the amount of material needed to create each pack of erasers.