Question

The sum of two numbers is 71. The difference of the two numbers is 35. What are the two numbers Let x be the larger number and y be the smaller number.

Answer 1

GIven:

Sum of two numbers is 71.

Difference of two number is 35.

x is larger than y.

Consider the two numbers as x and y. The two equations can be represented as.

`\begin{gathered} x+y=71\ldots\ldots\ldots.(1) \\ x-y=35\ldots\ldots\ldots.(2) \end{gathered}`

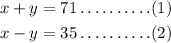
On solving equation (1) and (2),

`\begin{gathered} 2x=106 \\ x=(106)/(2) \\ x=53 \end{gathered}`

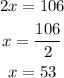
Now, substitute the value of x in equation (1).

`\begin{gathered} 53+y=71 \\ y=71-53 \\ y=18 \end{gathered}`

Hence, the value of x is 53 and the value of y is 18.