Question

Given p(x)= \frac{1}{ \sqrt[]{x} } and m(x)=x^2-4 find the indicated composition of functions. In addition to finding the three compositions also state the domain of each of the following 3 compositions using interval notation. You may want to do the work on paper and submit an image of that written work rather than try to type it all out.\frac{p(x)}{ m(x)}p(m(x))m(p(x))

Answer 1

We have the next function

`p(x)=\frac{1}{\sqrt[]{x}}`
`m(x)=x^2-4`

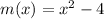
For the 1.

`(p(x))/(m(x))=\frac{\frac{1}{\sqrt[]{x}}}{x^2-4}`

We simplify

`(p(x))/(m(x))=\frac{1}{\sqrt[]{x}(x^2-4)}`

The domain is the set of all possible values that x can have in this case the domain is

`\: \mleft(0,\: 2\mright)\cup\mleft(2,\: \infty\: \mright)`

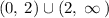
The range is the set of possible values that the function can have in this case the range is

`\: \: (-\infty\: ,\: -\frac{5\sqrt[4]{5}}{16√(2)}\rbrack\cup\mleft(0,\infty\mright)`

`(-\infty\: ,\: -0.3304\rbrack\cup(0,\: \infty\: )`

For 2.

`p(m(x))=\frac{1}{\sqrt[]{x^2-4}}`

We need to remember that we can have negative values inside a square root and we can divide between 0, therefore the domain is

`\mleft(-\infty\: ,\: -2\mright)\cup\mleft(2,\: \infty\: \mright)`

the range is

`\mleft(0,\: \infty\: \mright)`

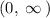
For 3.

`m(p(x))=(\frac{1}{\sqrt[]{x}})^2-4`

we simplify

`m(p(x))=(1)/(x)-4`

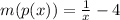
We need to remember the fact that we can divide between 0 therefore the domain is

`\: \mleft(-\infty\: ,\: 0\mright)\cup\mleft(0,\: \infty\: \mright)`

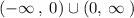
For the range we have

`\: \mleft(-\infty\: ,\: -4\mright)\cup\mleft(-4,\: \infty\: \mright)`