Question

Use slope to determine if lines AB and CD are parallel, perpendicular, or neither (-4, 7), B(-2, 6), C(2,-2), D(-8,3)

Answer 1

Parallel

Step-by-step explanation:

First, we find the slope of the line joining points A(-4, 7) and B(-2, 6).

`\begin{gathered} \text{Slope=}\frac{\text{Change in y}}{Change\text{ in x}} \\ =(6-7)/(-2-(-4)) \\ =(-1)/(-2+4) \\ =-(1)/(2) \end{gathered}`

Next, we find the slope of the line joining points C(2,-2) and D(-8,3).

`\begin{gathered} \text{Slope=}\frac{\text{Change in y}}{Change\text{ in x}} \\ =(3-(-2))/(-8-2) \\ =(5)/(-10) \\ =-(1)/(2) \end{gathered}`

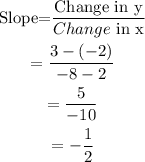
Since the slopes of the two lines are the same, lines AB and CD are parallel,