Question

The center pole of a tent is 8 feet long, and a side of the tent is 16 feetlong as shown in the diagram below. If a right angle is formed where thepole meets the ground, what is the measure of angle A to the nearestdegree?16 ft8 ft

Answer 1

The measure of angle A to the nearest degree is 30°

Step-by-step explanation:

The triangle is right angled, so we would apply trigonometry ratio SOHCAHTOA

Angle = A

opposite = side opposite angle A = 8 feet

hypotenuse = longest side = 16 ft

adjacent = base = ?

Now, since we know the opposie and hypotenuse, we would use sin ratio (SOH):

`\sin A=(opposite)/(hypotenuse)`
`\begin{gathered} \sin \text{ A = }\frac{8\text{ f}eet}{16\text{ f}et} \\ \sin \text{ A =}\frac{\text{ 1}}{2} \end{gathered}`

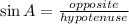
Take inverse of sine:

`\begin{gathered} A=sin^(-1)((1)/(2)) \\ A\text{ = }sin^(-1)(0.5) \\ A=\text{ 30}\degree \end{gathered}`

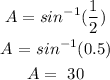
The measure of angle A to the nearest degree is 30°