The side lengths of a triangle are 5 cm, 6 cm, and 8 cm.
We are asked to determine whether the triangle is a right triangle or not.
Recall that a right triangle follows the Pythagorean theorem given by

Where a and b are the shorter sides and c is the longest side.
c = 8 since it is the longest side.
Substitute the values into the above equation and check if the equation is satisfied or not.
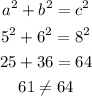
As you can see, the equation is not satisfied since 61 is not equal to 64.
Therefore, the given triangle is not a right triangle.