1 Answer

Buttonsrtoys · Answer 1 · 2023-06-27T01:34:28+0000

We have a line, y = -4x + 3, which is the image of a line k after the translation (x, y) --> (x+3, y-4).

We can apply this transformation to a line y = mx +b and then compare it to y = -4x + 3.

Wethen apply the transformation as:

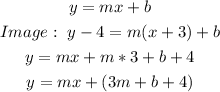
$\begin{gathered} y=mx+b \\ Image:\text{ }y-4=m(x+3)+b \\ y=mx+m*3+b+4 \\ y=mx+(3m+b+4) \end{gathered}$

We can now equal the slope and the y-intercept as:

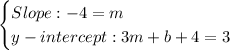
$\begin{cases}Slope:-4=m \\ y-intercept:3m+b+4=3\end{cases}$

The slope of k is m = -4 and the y-intercept can be calculated as:

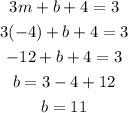
$\begin{gathered} 3m+b+4=3 \\ 3(-4)+b+4=3 \\ -12+b+4=3 \\ b=3-4+12 \\ b=11 \end{gathered}$

Then, as m = -4 and b = 11, we can write line k as y = -4x + 11.

Please log in or register to add a comment.