Question

hello, my name is kealohi- I would appreciate it if you could help me with is assignment that is signed? thank you.the question is : Select AL the correct answer.Which of these relations are functions?Two more relationsThere’s 2 Circles X - circle one 0Y - circle two 0X bubble : 4 connects to 216 connect to -73 connects to -23-5 connects to 12Another option{(-5,-7), (-2,-7),(7,17), (-5,21)}Also, there’s another option i didn’t see until now!It’s like the 3rd option. So.X = 4 | -4 | 7 | -7 | -4 |Y = 3 | -2 | 11 | 5 | -5 |

Answer 1

First and second relations

We can use the vertical line test. It consists of drawing any vertical line on the graph of the relation.

• If the line passes through two points or more, the relation is not a function.

,

• If the line passes only through one point, the relation is a function.

Graphically,

First relation

Since the line passes only through one point, then this relation is a function.

Second relation

Since the line passes through two points, then this relation is not a function.

Third relation

On the other hand, a relation is a function if the x-values map to only one y-value.

In this case, we can see that there are two different values of y when x = 2, that is:

`\begin{gathered} (x,y) \\ (2,11) \\ (2,15) \end{gathered}`

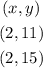
Since the x-values do not map to only one y-value, this relation is not a function.

Fourth relation

Again, a relation is a function if the x-values map to only one y-value. In this case, we can see that each element of the set of x-values connects one by one with elements of the set of y-values.

Therefore, this relation is a function.

Fifth relation

In this case, we can see that there are two different values of y when x = -5, that is:

`\begin{gathered} (x,y) \\ (-5,-7) \\ (-5,21) \end{gathered}`

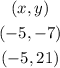
Since the x values do not map to only one y-value, this relation is not a function.

Sixth relation

In this case, we can see that there are two different values of y when x = -4, that is:

`\begin{gathered} (x,y) \\ (-4,-2) \\ (-4,-5) \end{gathered}`

Since the x values do not map to only one y-value, this relation is not a function.

In summary, we have:

• First relation: Is a function.

,

• Second relation: Is not a function.

,

• Third relation: Is not a function.

,

• Fourth relation: Is not a function.

,

• Fifth relation: Is not a function.

,

• Sixth relation: Is not a function.