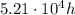Question

13. Earth is approximately 93,000,000 miles from the Sun, and Jupiter is approximately484,000,000 miles from the Sun. Assume that Sun, Earth and Jupiter are on the same straightline:Sun1JupiterEarthSunHow long would it take the Vikranta spaceship traveling at 7,500 mph to fly from Earth toJupiter? Express the answer in scientific notation to two decimal places. (5 points) (Hint: Findthe distance from Earth to Jupiter, then use 1 = dl r where t is time, d is distance and ris rate)r

Answer 1

So we need to find how long it would take the spaceship to travel from the Earth to Jupiter. This time is given by the distance between the Earth and Jupiter divided by the speed (rate) of the spaceship:

`t=(d)/(r)`

We already have the rate but we need to find the distance from the Earth to Jupiter. In order to do this we can use the distances from Earth to the Sun and from Jupiter to the Sun. The difference between these two is equal to the distance between the Earth and Jupiter. Then we have:

`d=484000000mi-93000000mi=391000000mi`

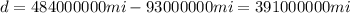
Knowing that the rate is 7500 mph we can find t:

`t=(391000000mi)/(7500(mi)/(h))=(391000000)/(7500)\cdot(mi)/((mi)/(h))=52133.333h`

So the time it would take is 52133.333h but we need to express it in scientific notation to two decimal places. Let's take this value and divide it by 10000:

`(52133.333h)/(10000)=5.2133333h`

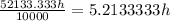
Remember that:

`10000=10^4`

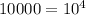
Then we get:

`(52133.333h)/(10000)=(52133.333h)/(10^4)=5.2133333h`

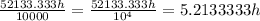
If we multiply both sides by 10^4 we get:

`\begin{gathered} (52133.333h)/(10^4)\cdot10^4=5.2133333h\cdot10^4 \\ 52133.333h=5.2133333\cdot10^4h \end{gathered}`

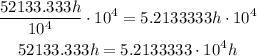
If we round the number in the right side to two decimal places we get the answer:

`5.21\cdot10^4h`