Let, Matt's age be x years and Emily's age be y years.
Matt is 9 years older than twice Emily’s age .
It can be written as,

And sum of their ages is 90

Put the value of x from equation (1) in equation (2).
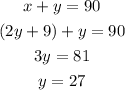
Put the value of y in equation (1),
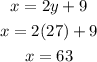
Hence, the Matt's age is x= 63 years and Emily's age is y=27 years.