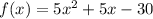
Step-by-step explanation
Step 1
the standard form of a quadratic equation is given by

then, by the table, we have:

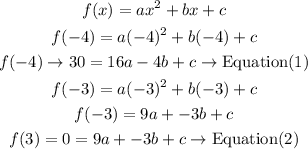
now, we have 2 equations, and 3 variables ( a, b and c, so we need one more equation)
when x= 2

Step 3
solve the equations
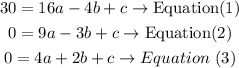
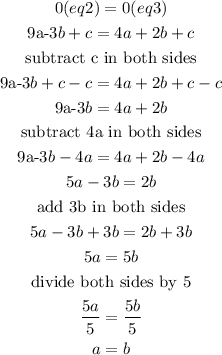
Now, replace a=b in equation (1) and equation (2)
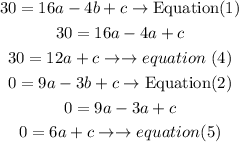
Step 3
use equations (4) and (5) to find a and c
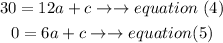
a)isolate c in both equations and equal the expressions to find a

we have a= 5,
now, replace in equation (4) to find x
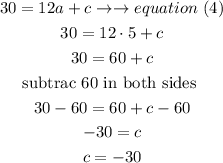
Therefore we have
a=5 b=5 c=-30
Step 4
finally, rewrite the equation
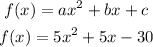
I hope this helps you