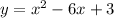We are asked to determine a quadratic equation given a set of points. To do that, let's remember that a quadratic function is of the following form:
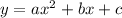
Now we replace the given points:
Replacing (3, -6)
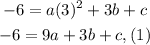
Replacing (1, -2)
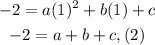
Replacing (6, 3)
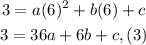
We get a system of three equations and three variables, these are:

Solving for "c" in equation (1):
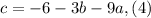
Solving for "c" in equation (2):
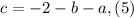
Solving for "c" in equation (3)
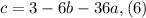
equating equations (4) and (5):
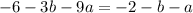
Rewritting:
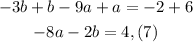
equating equation (5) and (6):
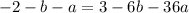
Rewritting:
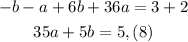
Now we solve for "a" in equation (7):

Replacing the value of 2a" in equation (8)
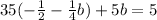
Solving the operations:
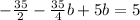
Solving for "b":

Adding 7/2 to both sides:
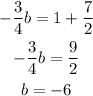
Therefore, b = 9.
Replacing this value in equation (7)
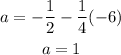
Replacing in equation (2)
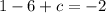
Adding like terms:
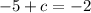
Adding 5 to both sides:
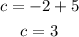
Therefore, the quadratic equation is: