Question

Y=5575•(0.65)^ta. What is the initial value? b. What is the decay factor?

Answer 1

Answer:

a) Initial Value = 5575

Decay factor = 0.35

Step-by-step explanation:

The given expression is:

`Y\text{ = 5575}*(0.65)^t`

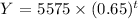
a) What is the initial value

The initial value of Y will take place when t = 0

Substituting t = 0 into the given equation:

`Y\text{ = 5575}*(0.65)^0`
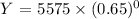
`Y=\text{ 5575 }*\text{ 1 = 5575}`The initial value is Y = 5575

b) What is the decay factor

The value of a substance due to an exponential decay is given by the general expression:

`Y=A(1-r)^t`

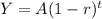
Where A = original/Initial value, and r = decay rate

Comparing the above equation with the given equation shown below:

`Y=5575(0.65)^t`

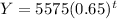
It is clear from the comparison of both equations that A = 5575 and :

1 - r = 0.65Solving for r:r = 1 - 0.65r = 0.35The decay factor is 0.35