ANSWER
Neither
Step-by-step explanation
To determine whether WX and YZ are parallel or perpendicular, wehave to find their slopes and compare them.
Slope is given as:
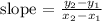
SLOPE OF WX
For WX,
(x1, y1) = (6, -6)
(x2, y2) =(7, 1)
So:
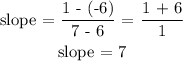
SLOPE OF YZ
For YZ:
(x1, y1) = (3, -1)
(x2, y2) = (2, 6)
So:
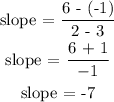
For WX and YZ to be parallel, their slope must be the same.
For WX and YZ to be perpendicular, the slope of one must be the negative inverse of the other.
Since their slopes are neither the same or the negative inverse of one another, WX and YX are neither parallel or perpendicular to one another.