Step-by-step explanation
So there are 24 donuts in the box with 3 different fillings. The probability of selecting a a jelly-filled donut first is given by the following quotient:
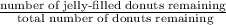
Since this is the first donut there are 2 jelly-filled donuts out of a total of 24. Then the probability of selecting a jelly-filled donut first is:
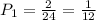
In a similar way we can find the probability of selecting a a custard-filled donut after selecting a jelly-filled donut in the first place. After the first selection we have 13 custard-filled donuts out of a total of 23 since one was already taken. Then this probability is:
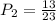
Finally, the probability of selecting a jelly-filled donut followed by a custard-filled donut is given by the product of the two probabilities we found:
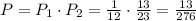
Answer
Then the answer is 13/276.