Given:
The tallest living man at one time had a height of 233 cm. The shortest living man at that time had a height of 74.5 cm. Heights of men at that time had a mean of 176.75 cm and a standard deviation of 6.24 cm.
Required:
We need to find that which man had the height that was more extreme
Step-by-step explanation:
The formula for z-score is
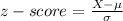
where

put the values for tallest
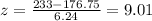
now for shortest

more the z-score more will be the extreme.
Final answer:
z-score for talles is 9.01
z-score for shortest is -16.39
and the extreme is tallest men