Question

Write an equation for the line parallel to g(t) = -5x - 1 and passing through the point (1,6). Write the answer in slope-intercept form.

Answer 1

The function given in the question is

`g(x)=-5x-1`

Question is to find the equation parallel to the line above and that passes through the point

`(1,6)`

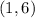
The general equation of a line in slope-intercept form is

`y=mx+c`

Where,

`\begin{gathered} m=\text{slope} \\ c=\text{intercept} \end{gathered}`

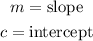
By comparing coefficients, we will have

`m=-5,c=-1`

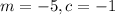
For two lines to be parallel, they must have the same value of their slopes

`m_1=m_2=-5`

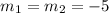
The formula used to calculate the equation of a line when the slope and the points are given is

`\begin{gathered} m=(y-y_1)/(x-x_1) \\ \text{where,} \\ m=-5,x_1=1,y_1=6 \end{gathered}`

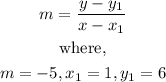
By substituting the values, we will have

`\begin{gathered} m=(y-y_1)/(x-x_1) \\ -5=(y-6)/(x-1) \end{gathered}`

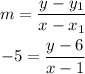
By cross multiplying the equation above, we will have

`\begin{gathered} -5=(y-6)/(x-1) \\ y-6=-5(x-1) \\ y-6=-5x+5 \\ \text{collect like terms, we will have} \\ y=-5x+5+6 \\ y=-5x+11 \end{gathered}`

Therefore,

The final answer is y = -5x + 11