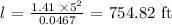Question

The electrical resistance of a wire varies directly with the length of the wire and inversely with the square of the diameter of the wire. If a wire 432 feet long and 4 millimeters in diameter has a resistance of 1.26 ohms, find the length of a wire of the same material whose resistance is 1.41 ohms and whose diameter is 5 millimeters.

Answer 1

`754.8\text{ ft}`

Step-by-step explanation:

We start by assigning variables to the measurements

Let us represent the resistance with r ,the length with l and diameter with d

The resistance is said to vary directly with the length and inversely with the square of the diameter of the wire

Mathematically, we have that as:

`r\text{ = }(kl)/(d^2)`

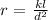
where k is the constant of proportionality

Now, let us get the value of k with the first set of values

We have:

l = 432 ft

d = 4 mm

r = 1.26 ohms

Substituting these values:

`\begin{gathered} 1.26\text{ = }\frac{k*\text{ 432}}{4^2} \\ \\ k\text{ = 0.0467 ohms mm/ft}^2 \end{gathered}`

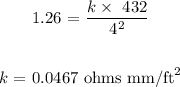
With this, we can now get the length of the wire

We can rearrange the formula in terms of l as follows:

`l\text{ = }(rd^2)/(k)`

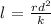
In this case:

r = 1.41 ohms

d = 5 mm

k is as calculated above

Substituting the values, we have that as:

`l\text{ = }\frac{1.41\text{ }*5^2}{0.0467}\text{ = 754.82 ft}`