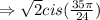The complex number to find the 4th root is given to be:

Step 1: Transform the complex number in the r.cisθ form
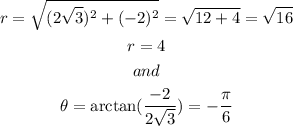
Therefore, the polar form is:
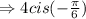
Step 2: According to the De Moivre's Formula, all n-th roots of a complex number
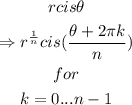
Therefore, the roots are as follows.
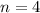
k = 0
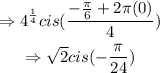
k = 1

k = 2
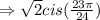
k = 3